You can navigate through this E-Book using the menu to the left. For E-Books that have a Chapter/Section organization each option in the menu to the left indicates a chapter and will open a menu showing the sections in that chapter. Alternatively, you can navigate to the next/previous section or chapter by clicking the links in the boxes at the very top and bottom of the material.
Also, depending upon the E-Book, it will be possible to download the complete E-Book, the chapter containing the current section and/or the current section. You can do this be clicking on the E-Book, Chapter, and/or the Section link provided below.
For those pages with mathematics on them you can, in most cases, enlarge the mathematics portion by clicking on the equation. Click the enlarged version to hide it.
In this section we’re going to make sure that you’re
familiar with functions and function notation.
Both will appear in almost every section in a Calculus class and so you
will need to be able to deal with them.
First, what exactly is a function? An equation will be a function if for any x in the domain of the equation (the
domain is all the x’s that can be
plugged into the equation) the equation will yield exactly one value of y.
This is usually easier to understand with an example.
|
Example 1 Determine
if each of the following are functions.
(a) 
(b)
Solution
(a) This first
one is a function. Given an x there is only one way to square it
and then add 1 to the result and so no matter what value of x you put into the equation there is
only one possible value of y.
(b) The only difference between this equation
and the first is that we moved the exponent off the x and onto the y. This small change is all that is required,
in this case, to change the equation from a function to something that isn’t
a function.
To see that this isn’t a function is fairly simple. Choose a value of x, say x=3 and plug
this into the equation.
Now, there are two possible values of y that we could use here.
We could use or . Since there are two possible values of y that we get from a single x this equation isn’t a function.
Note that this only needs to be the case for a single
value of x to make an equation not
be a function. For instance we could
have used x=-1 and in this case we
would get a single y (y=0).
However, because of what happens at x=3 this equation will not be a function.
|
Next we need to take a quick look at function notation. Function notation is nothing more than a
fancy way of writing the y in a
function that will allow us to simplify notation and some of our work a little.
Let’s take a look at the following function.
Using function notation we can write this as any of the
following.
Recall that this is NOT a letter times x, this is just a fancy way of writing y.
So, why is this useful?
Well let’s take the function above and let’s get the value of the
function at x=-3. Using function notation we represent the
value of the function at x=-3 as f(-3).
Function notation gives us a nice compact way of representing function
values.
Now, how do we actually evaluate the function? That’s really simple. Everywhere we see an x on the right side we will substitute whatever is in the
parenthesis on the left side. For our
function this gives,
Let’s take a look at some more function evaluation.
|
Example 2 Given
find each of the following.
(a) [Solution]
(b) [Solution]
(c) [Solution]
(d) [Solution]
(e) [Solution]
(f) [Solution]
Solution
(a)
[Return to Problems]
(b)
Be
careful when squaring negative numbers!
[Return to Problems]
(c)
Remember that we substitute for the x’s WHATEVER is in the parenthesis on the left. Often this will be something other than a
number. So, in this case we put t’s in for all the x’s on the left.
[Return to Problems]
(d)
Often instead of evaluating functions at numbers or single
letters we will have some fairly complex evaluations so make sure that you
can do these kinds of evaluations.
[Return to Problems]
(e)
The only difference between this one and the previous one
is that I changed the t to an x.
Other than that there is absolutely no difference between the
two! Don’t get excited if an x appears inside the parenthesis on
the left.
[Return to Problems]
(f)
This one is not much different from the previous
part. All we did was change the
equation that we were plugging into function.
[Return to Problems]
|
All throughout a calculus course we will be finding roots of
functions. A root of a function is
nothing more than a number for which the function is zero. In other words, finding the roots of a
function, g(x), is equivalent to
solving
|
|
|
|
|
Example 3 Determine
all the roots of
Solution
So we will need to solve,
First, we should factor the equation as much as
possible. Doing this gives,
Next recall that if a product of two things are zero then
one (or both) of them had to be zero.
This means that,
From the first it’s clear that one of the roots must then
be t=0. To get the remaining roots we will need to
use the quadratic formula on the second equation. Doing this gives,
In order to remind you how to simplify radicals we gave
several forms of the answer.
To complete the problem, here is a complete list of all
the roots of this function.
Note we didn’t use the final form for the roots from the
quadratic. This is usually where we’ll
stop with the simplification for these kinds of roots. Also note that, for the sake of the
practice, we broke up the compact form for the two roots of the
quadratic. You will need to be able to
do this so make sure that you can.
|
This example had a couple of points other than finding roots
of functions.
The first was to remind you of the quadratic formula. This won’t be the last time that you’ll need it in this class.
The second was to get you used to seeing “messy”
answers. In fact, the answers in the
above list are not that messy. However,
most students come out of an Algebra class very used to seeing only integers
and the occasional “nice” fraction as answers.
So, here is fair warning.
In this class I often will intentionally make the answers look “messy”
just to get you out of the habit of always expecting “nice” answers. In “real life” (whatever that is) the answer
is rarely a simple integer such as two.
In most problems the answer will be a decimal that came about from a
messy fraction and/or an answer that involved radicals.
One of the more important ideas about functions is that of
the domain and range of a function. In
simplest terms the domain of a function is the set of all values that can be
plugged into a function and have the function exist and have a real number for
a value. So, for the domain we need to
avoid division by zero, square roots of negative numbers, logarithms of zero
and negative numbers (if not familiar with logarithms we’ll take a look at them
in a little later), etc. The range of a function
is simply the set of all possible values that a function can take.
Let’s find the domain and range of a few functions.
|
Example 4 Find
the domain and range of each of the following functions.
(a) [Solution]
(b) [Solution]
(c) [Solution]
(d) [Solution]
(e) [Solution]
Solution
(a)
We know that this is a line and that it’s not a horizontal
line (because the slope is 5 and not zero…).
This means that this function can take on any value and so the range is
all real numbers. Using “mathematical”
notation this is,
This is more
generally a polynomial and we know that we can plug any value into a
polynomial and so the domain in this case is also all real numbers or,
[Return to Problems]
(b)
This is a square root and we know that square roots are
always positive or zero and because we can have the square root of zero in
this case,
We know then
that the range will be,
For the domain
we have a little bit of work to do, but not much. We need to make sure that we don’t take
square roots of any negative numbers and so we need to require that,
The domain is
then,
[Return to Problems]
(c)
Here we have a quadratic which is a polynomial and so we
again know that the domain is all real numbers or,
In this case the range requires a little bit of work. From an Algebra class we know that the
graph of this will be a parabola that
opens down (because the coefficient of the is negative) and so the vertex will be the
highest point on the graph. If we know
the vertex we can then get the range.
The vertex is then,
So, as
discussed, we know that this will be the highest point on the graph or the
largest value of the function and the parabola will take all values less than
this so the range is then,
[Return to Problems]
(d)
This function contains an absolute value and we know that
absolute value will be either positive or zero. In this case the absolute value will be
zero if and so the absolute value portion of this
function will always be greater than or equal to zero. We are subtracting 3 from the absolute
value portion and so we then know that the range will be,
We can plug any
value into an absolute value and so the domain is once again all real numbers
or,
[Return to Problems]
(e)
This function may seem a little tricky at first but is
actually the easiest one in this set of examples. This is a constant function and so an value
of x that we plug into the function
will yield a value of 8. This means
that the range is a single value or,
The domain is
all real numbers,
[Return to Problems]
|
In general determining the range of a function can be
somewhat difficult. As long as we
restrict ourselves down to “simple” functions, some of which we looked at in
the previous example, finding the range is not too bad, but for most functions
it can be a difficult process.
Because of the difficulty in finding the range for a lot of
functions we had to keep those in the previous set somewhat simple, which also
meant that we couldn’t really look at some of the more complicated domain
examples that are liable to be important in a Calculus course. So, let’s take a look at another set of
functions only this time we’ll just look for the domain.
|
Example 5 Find
the domain of each of the following functions.
(a) [Solution]
(b) [Solution]
(c) [Solution]
Solution
(a)
Okay, with this problem we need to avoid division by zero
and so we need to determine where the denominator is zero which means
solving,
So, these are
the only values of x that we need
to avoid and so the domain is,
[Return to Problems]
(b)
In this case we need to avoid square roots of negative
numbers and so need to require that,
Note that we
multiplied the whole inequality by -1 (and remembered to switch the direction
of the inequality) to make this easier to deal with. You’ll need to be able to solve
inequalities like this more than a few times in a Calculus course so let’s
make sure you can solve these.
The first thing
that we need to determine where the function is zero and that’s not too
difficult in this case.
So, the
function will be zero at and . Recall that these points will be the only
place where the function may change
sign. It’s not required to change sign
at these points, but these will be the only points where the function can
change sign. This means that all we
need to do is break up a number line into the three regions that avoid these
two points and test the sign of the function at a single point in each of the
regions. If the function is positive
at a single point in the region it will be positive at all points in that
region because it doesn’t contain the any of the points where the function
may change sign. We’ll have a similar
situation if the function is negative for the test point.
So, here is a
number line showing these computations.
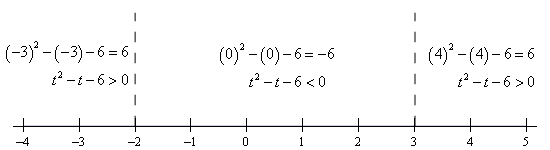
From this we
can see that in only region in which the quadratic (in its modified form) will
be negative is in the middle region.
Recalling that we got to the modified region by multiplying the
quadratic by a -1 this means that the quadratic under the root will only be
positive in the middle region and so the domain for this function is then,
[Return to Problems]
(c)
In this case we have a mixture of the two previous
parts. We have to worry about division
by zero and square roots of negative numbers.
We can cover both issues by requiring that,
Note that we
need the inequality here to be strictly greater than zero to avoid the
division by zero issues. We can either
solve this by the method from the previous example or, in this case, it is
easy enough to solve by inspection.
The domain is this case is,
[Return to Problems]
|
The next topic that we need to discuss here is that of function composition. The composition of f(x) and g(x) is
In other words, compositions are evaluated by plugging the
second function listed into the first function listed. Note as well that order is important
here. Interchanging the order will usually
result in a different answer.
|
Example 6 Given
and find each of the following.
(a) [Solution]
(b) [Solution]
(c) [Solution]
(d) [Solution]
Solution
(a)
In this case we’ve got a number instead of an x but it works in exactly the same
way.
[Return to Problems]
(b)
Compare this answer to the next part and notice that
answers are NOT the same. The order in
which the functions are listed is important!
[Return to Problems]
(c)
And just to make the point. This answer is different from the previous
part. Order is important in
composition.
[Return to Problems]
(d)
In this case do not get excited about the fact that it’s
the same function. Composition still
works the same way.
[Return to Problems]
|
Let’s work one more example that will lead us into the next
section.
In this case the two compositions where the same and in fact
the answer was very simple.
This will usually not happen. However, when the two compositions are the
same, or more specifically when the two compositions are both x there is a very nice relationship
between the two functions. We will take
a look at that relationship in the next section.