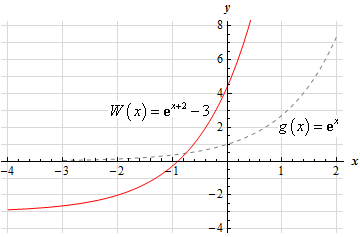The Algebraic transformations we were using in the first few problems of this section can be combined to shift a graph up/down and right/left at the same time. If we know the graph of $g\left( x \right)$ then the graph of $g\left( {x + c} \right) + k$ is simply the graph of $g\left( x \right)$ shifted right by $c$ units if $c < 0$ or shifted left by $c$ units if $c > 0$ and shifted up by $k$ units if $k > 0$ or shifted down by $k$ units if $k < 0$.

So, in our case if $g\left( x \right) = {{\bf{e}}^x}$ we can see that,

and so the graph we’re being asked to sketch is the graph of $g\left( x \right) = {{\bf{e}}^x}$shifted left by 2 units and down by 3 units.

Here is the graph of $W\left( x \right) = {{\bf{e}}^{x + 2}} - 3$ and note that to help see the transformation we have also sketched in the graph of $g\left( x \right) = {{\bf{e}}^x}$.

In this case the resulting sketch of $W\left( x \right)$ that we get by shifting the graph of $g\left( x \right)$ is not really the best, as it pretty much cuts off at $x = 0$ so in this case we should probably extend the graph of $W\left( x \right)$ a little. Here is a better sketch of the graph.