Section 4.2 : Parabolas
6. Sketch the graph of the following parabola. The graph should contain the vertex, the \(y\)‑intercept, \(x\)-intercepts (if any) and at least one point on either side of the vertex.
\[f\left( x \right) = 4{x^2} - 4x + 1\]Show All Steps Hide All Steps
Start SolutionLet’s find the vertex first. In this case the equation is in the form \(f\left( x \right) = a{x^2} + bx + c\). And so we know the vertex is the point \(\left( { - \frac{b}{{2a}},f\left( { - \frac{b}{{2a}}} \right)} \right)\). The vertex is then,
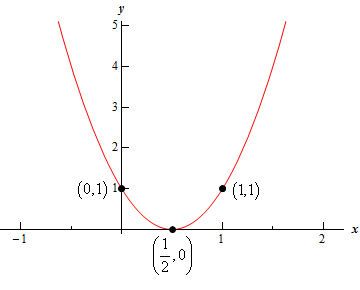
\[\left( { - \frac{{ - 4}}{{2\left( 4 \right)}},f\left( { - \frac{{ - 4}}{{2\left( 4 \right)}}} \right)} \right) = \left( {\frac{1}{2},f\left( {\frac{1}{2}} \right)} \right) = \left( {\frac{1}{2},0} \right)\]Also note that \(a = 4 > 0\) for this parabola and so the parabola will open upwards.
Show Step 2The \(y\)-intercept is just the point \(\left( {0,f\left( 0 \right)} \right)\). A quick function evaluation gives us that \(f\left( 0 \right) = 1\) and so for our equation the \(y\)-intercept is \(\left( {0,1} \right)\).
Show Step 3For the \(x\)-intercepts we would normally solve the equation \(f\left( x \right) = 0\). However, in this case we don’t need to do that. From the first step we see that the vertex has a y‑coordinate of zero and hence is also an \(x\)-intercept. Also, because it is the vertex this can be the only \(x\)-intercept for this function.
Note that if we’d solved the equation we would have also arrived at this single \(x\)‑intercept.
Show Step 4In this case all we have are the vertex (which also happens to be the single \(x\)‑intercept) and the \(y\)-intercept (which is on the right side of the vertex). So, we’ll need a point that is on the left side of the vertex and we can find the point on the left side of the vertex that corresponds to the \(y\)-intercept for this point.
The \(y\)-intercept is a distance of 1 to the left of the vertex and so there will be a corresponding point at the same \(y\) value to the right and it will be a distance of 1 to the right of the vertex. Therefore, the point to the right of the vertex corresponding to the y‑intercept is \(\left( {1,1} \right)\).
Show Step 5Here is a sketch of the parabola including all the points we found above.