We need the 1^st derivative to get the critical points so here it is.

\[\begin{align*}h'\left( t \right) & = 2t{\left( {t - 7} \right)^{{\frac{1}{3}}}} + {t^2}\left( {{\frac{1}{3}}} \right){\left( {t - 7} \right)^{ - \,\,{\frac{2}{3}}}} = 2t{\left( {t - 7} \right)^{{\frac{1}{3}}}} + \frac{{{t^2}}}{{3{{\left( {t - 7} \right)}^{{\frac{2}{3}}}}}}\\ & = \frac{{6t\left( {t - 7} \right) + {t^2}}}{{3{{\left( {t - 7} \right)}^{{\frac{2}{3}}}}}} = \frac{{7{t^2} - 42t}}{{3{{\left( {t - 7} \right)}^{{\frac{2}{3}}}}}} = \frac{{7t\left( {t - 6} \right)}}{{3{{\left( {t - 7} \right)}^{{\frac{2}{3}}}}}}\end{align*}\]

Now, recall that critical points are where the derivative doesn’t exist or is zero. Because we simplified and factored the derivative as much as possible we can clearly see that the derivative does not exist at \(t = 7\) (and the function exists here…) and that the derivative is zero at \(t = 0\) and \(t = 6\). The critical points of this function are then,

\[\require{bbox} \bbox[2pt,border:1px solid black]{{t = 0,\,\,\,\,\,\,t = 6,\,\,\,\,\,\,t = 7}}\]

To determine the increase/decrease information for the function all we need is a quick number line for the derivative. Here is the number line.

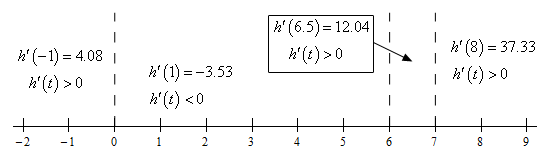
From this we get the following increasing/decreasing information for the function.

\[\require{bbox} \bbox[2pt,border:1px solid black]{{{\mbox{Increasing : }}\left( { - \infty ,0} \right),\,\,\,\,\,\,\left( {6,7} \right)\,\,\,\,\& \,\,\,\left( {7,\infty } \right)\hspace{0.25in}\hspace{0.25in}{\mbox{Decreasing : }}\,\,\left( {0,6} \right)}}\]

With the increasing/decreasing information from the previous step we can easily classify the critical points using the 1^st derivative test. Here is classification of the functions critical points.

\[\require{bbox} \bbox[2pt,border:1px solid black]{\begin{align*}t & = 0\,\,\,\,\,\,:\,{\mbox{ Relative Maximum}}\\ t & = 6\,\,\,\,\,\,:\,{\mbox{ Relative Minimum}}\\ t & = 7\,\,\,\,\,\,:\,{\mbox{ Neither}}\end{align*}}\]