Section 15.2 : Iterated Integrals
9. Determine the volume that lies under \(f\left( {x,y} \right) = 9{x^2} + 4xy + 4\) and above the rectangle given by \(\left[ { - 1,1} \right] \times \left[ {0,2} \right]\) in the \(xy\)-plane.
Show All Steps Hide All Steps
Start SolutionFirst, let’s start off with a quick sketch of the function.
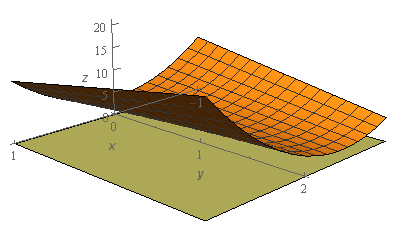
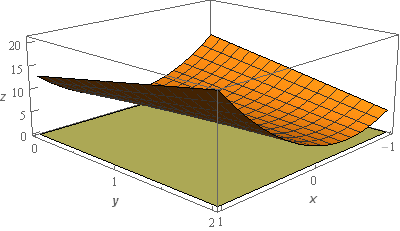
The greenish rectangle under the surface is \(R\) from the problem statement and is given to help visualize the relationship between the surface and \(R\).
Note that the sketch isn’t really needed for this problem. It is just here so we can get a feel for what the problem is asking of us. Also, the sketch is presented in two ways to help “see” the surface. Sometimes it is easier to see what is going on with both a “standard” set of axes and a “box frame” set of axes.
Now, we know from the notes that the volume is given by,
\[V = \iint\limits_{R}{{9{x^2} + 4xy + 4\,dA}}\]where \(R\) is the rectangle given in the problem statement.
Show Step 2Now, as with the other problems in this section we need to determine the order of integration. In this case there doesn’t seem to be much difference between the two so we’ll integrate with respect to \(x\) first simply because there are more of them and so maybe we’ll “simplify” the integral somewhat after doing that integration.
Here is the \(x\) integration work.
\[\begin{align*}V & = \iint\limits_{R}{{9{x^2} + 4xy + 4\,dA}}\\ & = \int_{0}^{2}{{\int_{{ - 1}}^{1}{{9{x^2} + 4xy + 4\,dx}}\,dy}} = \int_{0}^{2}{{\left. {\left( {3{x^3} + 2{x^2}y + 4x} \right)} \right|_{ - 1}^1\,dy}} = \int_{0}^{2}{{14\,dy}}\end{align*}\]In this case the second term involving the \(y\) actually canceled out when doing the \(x\) limit evaluation. This will happen on occasion and isn’t something we should get excited about when it happens.
Show Step 3Finally, all we need to do is the very simply \(y\) integration. Here is that work.
\[V = \int_{0}^{2}{{14\,dy}} = \left. {\left( {14y} \right)} \right|_0^2 = \require{bbox} \bbox[2pt,border:1px solid black]{{28}}\]