Section 16.4 : Line Integrals of Vector Fields
7. Evaluate \( \displaystyle \int\limits_{C}{{\vec F\centerdot d\vec r}}\) where \(\vec F\left( {x,y} \right) = \left( {6x - 2y} \right)\,\vec i + {x^2}\vec j\) for each of the following curves.
- \(C\) is the line segment from \(\left( {6, - 3} \right)\) to \(\left( {0,0} \right)\) followed by the line segment from \(\left( {0,0} \right)\) to \(\left( {6,3} \right)\).
- \(C\) is the line segment from \(\left( {6, - 3} \right)\) to \(\left( {6,3} \right)\).
Start Solution
Let’s start off with a quick sketch of the curve for this part of the problem.
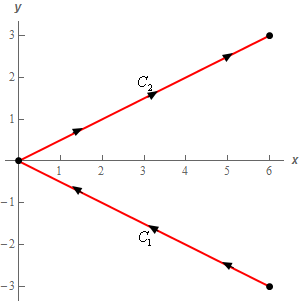
Here is the parameterization for each of these curves.
\({C_1}:\,\,\vec r\left( t \right) = \left( {1 - t} \right)\left\langle {6, - 3} \right\rangle + t\left\langle {0,0} \right\rangle = \left\langle {6 - 6t, - 3 + 3t} \right\rangle \hspace{0.25in}\,\,\,\,\,\,\,0 \le t \le 1\)\({C_2}:\,\,\vec r\left( t \right) = \left\langle {t,\frac{1}{2}t} \right\rangle \hspace{0.25in}\,\,\,\,\,\,\,0 \le t \le 6\)
For \({C_2}\) we used the equation of the line to get the parameterization because it gave a slightly nicer form to work with. We couldn’t do this with \({C_1}\) because the specified direction of the curve was in the decreasing \(x\) direction and the limits of the integral need to be from smaller value to larger value.
Show Step 2Here is the line integral for each of these curves.
\[\begin{align*}\int\limits_{{{C_1}}}{{\vec F\centerdot d\vec r}} & = \int_{0}^{1}{{\left\langle {6\left( {6 - 6t} \right) - 2\left( { - 3 + 3t} \right),{{\left( {6 - 6t} \right)}^2}} \right\rangle \centerdot \left\langle { - 6,3} \right\rangle \,dt}}\\ & = \int_{0}^{1}{{252t - 252 + 3{{\left( {6 - 6t} \right)}^2}\,dt}}\\ & = \left. {\left( {126{t^2} - 252t - \frac{1}{6}{{\left( {6 - 6t} \right)}^3}} \right)} \right|_0^1 = \underline { - 90} \end{align*}\] \[\begin{align*}\int\limits_{{{C_2}}}{{\vec F\centerdot d\vec r}} & = \int_{0}^{6}{{\left\langle {6\left( t \right) - 2\left( {\frac{1}{2}t} \right),{{\left( t \right)}^2}} \right\rangle \centerdot \left\langle {1,\frac{1}{2}} \right\rangle \,dt}}\\ & = \int_{0}^{6}{{5t + \frac{1}{2}{t^2}\,dt}} = \left. {\left( {\frac{5}{2}{t^2} + \frac{1}{6}{t^3}} \right)} \right|_0^6 = \underline {126} \end{align*}\] Show Step 3The line integral for this part is then,
\[\int\limits_{C}{{\vec F\centerdot d\vec r}} = \left( { - 90} \right) + \left( {126} \right) = \require{bbox} \bbox[2pt,border:1px solid black]{{36}}\]b \(C\) is the line segment from \(\left( {6, - 3} \right)\) to \(\left( {6,3} \right)\). Show All Steps Hide All Steps
Start Solution
Let’s start off with a quick sketch of the curve for this part of the problem.
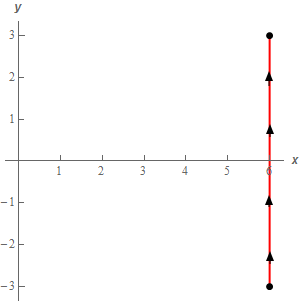
So, what we have in this part is a different curve that goes from \(\left( {6, - 3} \right)\) to \(\left( {6,3} \right)\). Despite the fact that this curve has the same starting and ending point as the curve in the first part there is no reason to expect the line integral to have the same value. Therefore, we’ll need to go through the work and see what we get from the line integral.
We’ll need to parameterize the curve so let’s take care of that.
\(C:\,\,\vec r\left( t \right) = \left\langle {6,t} \right\rangle \hspace{0.25in}\,\,\,\,\,\,\, - 3 \le t \le 3\)Show Step 2
Now all we need to do is compute the line integral.
\[\begin{align*}\int\limits_{C}{{\vec F\centerdot d\vec r}} & = \int_{{ - 3}}^{3}{{\left\langle {6\left( 6 \right) - 2\left( t \right),{{\left( 6 \right)}^2}} \right\rangle \centerdot \left\langle {0,1} \right\rangle \,dt}}\\ & = \int_{{ - 3}}^{3}{{36\,dt}} = \left. {\left( {36t} \right)} \right|_{ - 3}^3 = \require{bbox} \bbox[2pt,border:1px solid black]{{216}}\end{align*}\]So, as noted at the start of this part the value of the line integral was not the same as the value of the line integral in the first part despite the same starting and ending points for the curve. Note that it is possible for two line integrals with the same starting and ending points to have the same value but we can’t expect that to happen and so need to go through and do the work.