Section 15.7 : Triple Integrals in Spherical Coordinates
1. Evaluate ∭E10xz+3dV where E is the region portion of x2+y2+z2=16 with z≥0.
Show All Steps Hide All Steps
Start SolutionOkay, let’s start off with a quick sketch of the region E so we can get a feel for what we’re dealing with.
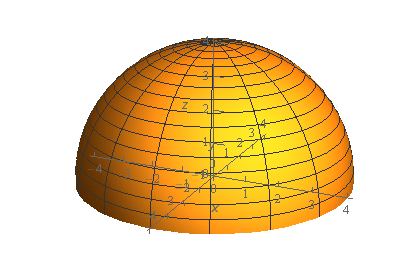

We’ve given the sketches with a set of “traditional” axes as well as a set of “box” axes to help visualize the surface and region.
In this case we’re dealing with the upper half of a sphere of radius 4.
Show Step 2Now, since we are integrating over a portion of a sphere it makes sense to use spherical coordinate for the integral and the limits are,
0≤φ≤π20≤θ≤2π0≤ρ≤4Remember that φ is the angle from the positive z-axis that we rotate through as we cover the region and θ is the angle we rotate around the z-axis as we cover the region.
In this case we have the full upper half of the sphere and so θ will range from 0 to 2π while φ will range from 0 to π2.
Show Step 3Plugging these limits into the integral and converting to spherical coordinates gives,
∭E10xz+3dV=∫π20∫2π0∫40[10(ρsinφcosθ)(ρcosφ)+3](ρ2sinφ)dρdθdφ=∫π20∫2π0∫4010ρ4sin2φcosφcosθ+3ρ2sinφdρdθdφDon’t forget to convert the x and z into spherical coordinates and also don’t forget that dV=ρ2sinφdρdθdφ and so we’ll pick up a couple of extra terms when converting the dV to spherical coordinates.
Show Step 4Okay, now all we need to do is evaluate the integral. Here is the ρ integration.
∭E10xz+3dV=∫π20∫2π0(2ρ5sin2φcosφcosθ+ρ3sinφ)|40dθdφ=∫π20∫2π02048sin2φcosφcosθ+64sinφdθdφ Show Step 5Next let’s do the θ integration.
∭E10xz+3dV=∫π20(2048sin2φcosφsinθ+64θsinφ)|2π0dφ=∫π20128πsinφdφ Show Step 6Finally, we’ll do the φ integration.
∭E10xz+3dV=(−128πcosφ)|π20=128πNote that, in this case, because the limits of each of the integrals were all constants we could have done the integration in any order we wanted to. In this case, it might have been “simpler” to do the φ first or second as that would have greatly reduced the integrand for the remaining integral(s).