Section 15.6 : Triple Integrals in Cylindrical Coordinates
2. Evaluate \(\displaystyle \iiint\limits_{E}{{{{\bf{e}}^{ - {x^{\,2}} - {z^{\,2}}}}\,dV}}\) where \(E\) is the region between the two cylinders \({x^2} + {z^2} = 4\) and \({x^2} + {z^2} = 9\) with \(1 \le y \le 5\) and \(z \le 0\).
Show All Steps Hide All Steps
Start SolutionOkay, let’s start off with a quick sketch of the region \(E\) so we can get a feel for what we’re dealing with.
We know that \({x^2} + {z^2} = 4\) and \({x^2} + {z^2} = 9\) are cylinders of radius 2 and 3 respectively that are centered on the \(y\)-axis. The range \(1 \le y \le 5\) tells us that we will only have the cylinders in this range of \(y\)’s. Finally, the \(z \le 0\) tells us that we will only have the lower half of each of the cylinders.
Here then is the sketch of \(E\).
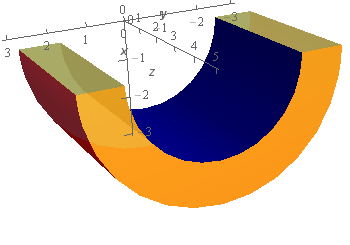

We’ve given the sketches with a set of “traditional” axes as well as a set of “box” axes to help visualize the surface and region.
The “front” of \(E\) is just the portion of the plane \(y = 5\) that “caps” the front and is the orange ring in the sketch. The “back” of \(E\) is the portion of the plane \(y = 1\) that caps the back of the region and is not shown in the sketch due to the orientation of axis system.
Show Step 2So, from the sketch above it looks like the region \(D\) will be back in the \(xz\)-plane and so we’ll need to integrate with respect to \(y\) first. In this case this is even easier because both the front and back portions of the surfaces are just the planes \(y = 5\) and \(y = 1\) respectively. That means that the \(y\) limits are,
\[1 \le y \le 5\] Show Step 3Now, let’s think about the \(D\) for this problem. If we look at the object from along the \(y\) axis we see the lower half of the ring with radii 2 and 3 as shown below.
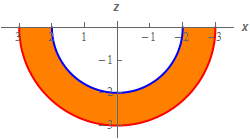
Note that the \(x\)-axis orientation is switched from the standard orientation to more accurately match what you’d see if you did look at \(E\) from along the \(y\)-axis. In other words, the positive \(x\) values are on the left side and the negative \(x\) values are on the right side. The orientation of the \(x\)-axis doesn’t change that we still see a portion of a ring that lies in the \(xz\)-plane and this is in fact the \(D\) for this problem.
Because \(D\) is in the \(xz\)-plane and it is a portion of a ring that means that we’ll need to use the following “modified” version of cylindrical coordinates.
\[\begin{align*}x & = r\cos \theta \\ y & = y\\ z & = r\sin \theta \end{align*}\]This also matches up with the fact that we need to integrate \(y\) first (as we determined in Step 2) and the first variable of integration with cylindrical coordinates is always the “free” variable (i.e. not the one involving the trig functions).
So, we can easily describe the ring in terms of \(r\) and \(\theta \) so here are the cylindrical coordinates for this problem.
\[\begin{array}{c}\pi \le \theta \le 2\pi \\ \,2 \le r \le 3\\ \,1 \le y \le 5\end{array}\] Show Step 4Plugging these limits into the integral and converting to cylindrical coordinates gives,
\[\iiint\limits_{E}{{{{\bf{e}}^{ - {x^{\,2}} - {z^{\,2}}}}\,dV}} = \int_{\pi }^{{2\pi }}{{\int_{2}^{3}{{\int_{1}^{5}{{r\,{{\bf{e}}^{ - \,\,{r^{\,2}}}}\,dy}}\,dr}}\,d\theta }}\]Don’t forget \({x^2} + {z^2} = {r^2}\) under our modified cylindrical coordinates and also don’t forget that \(dV = r\,dy\,dr\,d\theta \) and so we pick up another \(r\) when converting the \(dV\) to cylindrical coordinates (that will be very helpful with the \(r\) integration).
Show Step 5Okay, now all we need to do is evaluate the integral. Here is the \(y\) integration.
\[\begin{align*}\iiint\limits_{E}{{{{\bf{e}}^{ - {x^{\,2}} - {z^{\,2}}}}\,dV}} & = \int_{\pi }^{{2\pi }}{{\int_{2}^{3}{{\left. {r\,{{\bf{e}}^{ - \,\,{r^{\,2}}}}y} \right|_1^5\,dr}}\,d\theta }}\\ & = \int_{\pi }^{{2\pi }}{{\int_{2}^{3}{{4r\,{{\bf{e}}^{ - \,\,{r^{\,2}}}}\,dr}}\,d\theta }}\end{align*}\] Show Step 6Next let’s do the \(r\) integration.
\[\begin{align*}\iiint\limits_{E}{{{{\bf{e}}^{ - {x^{\,2}} - {z^{\,2}}}}\,dV}} & = \int_{\pi }^{{2\pi }}{{\left. {\left( { - 2\,{{\bf{e}}^{ - \,\,{r^{\,2}}}}} \right)} \right|_2^3\,d\theta }}\\ & = \int_{\pi }^{{2\pi }}{{2\left( {{{\bf{e}}^{ - 4}} - {{\bf{e}}^{ - 9}}} \right)\,d\theta }}\end{align*}\] Show Step 7Finally, we’ll do the \(\theta \) integration.
\[\iiint\limits_{E}{{{{\bf{e}}^{ - {x^{\,2}} - {z^{\,2}}}}\,dV}} = \left. {2\left( {{{\bf{e}}^{ - 4}} - {{\bf{e}}^{ - 9}}} \right)\theta} \right|_\pi ^{2\pi } = \require{bbox} \bbox[2pt,border:1px solid black]{{2\pi \left( {{{\bf{e}}^{ - 4}} - {{\bf{e}}^{ - 9}}} \right) = 0.1143}}\]The trickiest part of this one was probably the sketch of \(E\). Once you see that and how to get the \(D\) for the integral the rest of the problem was pretty simple for the most part.