Calculus III - Double Integrals over General Regions

Section 15.3 : Double Integrals over General Regions

7. Evaluate $ \displaystyle \iint\limits_{D}{{3 - 6xy\,dA}}$ where $D$ is the region shown below.

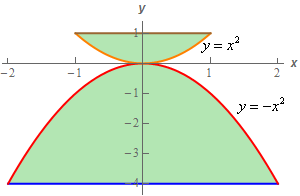
$The region $D$ is shaded in. It is bounded above by the horizontal line $y = 1$ and below by the horizontal line $y = - 4$. The upper part of the region is the area inside the upward opening parabola $y = {x^2}$, running from $\left( { - 1,1} \right)$ across to $\left( {1,1} \right)$, and the lower part is the area inside the downward opening parabola $y = - {x^2}$, running from $\left( { - 2, - 4} \right)$ across to $\left( {2, - 4} \right)$. The two parts meet at the origin where the region pinches down to a single point.$

Show All Steps Hide All Steps

Start Solution

First, let’s label the two sub regions in $D$ as shown below.

Show Step 2

Hopefully it is clear that we cannot get a single set of limits that will completely describe $D$ so we’ll need to split the integral as follows.

\[\iint\limits_{D}{{3 - 6xy\,dA}} = \iint\limits_{{{D_{\,1}}}}{{3 - 6xy\,dA}} + \iint\limits_{{{D_{\,2}}}}{{3 - 6xy\,dA}}\]

Show Step 3

The region should also (hopefully) make it clear that we’ll need to integrate $y$ first with both of the integrals. So, here are the limits for each integral.

\[\begin{array}{*{20}{c}}\begin{array}{c}{D_{\,1}}\,\\ - 1 \le x \le 1\\ {x^2} \le y \le 1\end{array}&{\hspace{0.5in}}&\begin{array}{c}{D_{\,2}}\\ - 2 \le x \le 2\\ - 4 \le y \le - {x^2}\end{array}\end{array}\]

The integrals are then,

\[\iint\limits_{D}{{3 - 6xy\,dA}} = \int_{{ - 1}}^{1}{{\int_{{{x^2}}}^{1}{{3 - 6xy\,dy}}\,dx}} + \int_{{ - 2}}^{2}{{\int_{{ - 4}}^{{ - {x^2}}}{{3 - 6xy\,dy}}\,dx}}\]

Show Step 4

Not much to do now other than do the integrals. Here is the $y$ integration for both of them.

\[\begin{align*}\iint\limits_{D}{{3 - 6xy\,dA}} & = \int_{{ - 1}}^{1}{{\left. {\left( {3y - 3x{y^2}} \right)} \right|_{{x^2}}^1\,dx}} + \int_{{ - 2}}^{2}{{\left. {\left( {3y - 3x{y^2}} \right)} \right|_{ - 4}^{ - {x^2}}\,dx}}\\ & = \int_{{ - 1}}^{1}{{3{x^5} - 3{x^2} - 3x + 3\,dx}} + \int_{{ - 2}}^{2}{{12 + 48x - 3{x^2} - 3{x^5}\,dx}}\end{align*}\]

Show Step 5

Finally, here is the $x$ integration for both of the integrals.

\[\begin{align*}\iint\limits_{D}{{3 - 6xy\,dA}} & = \int_{{ - 1}}^{1}{{3{x^5} - 3{x^2} - 3x + 3\,dx}} + \int_{{ - 2}}^{2}{{12 + 48x - 3{x^2} - 3{x^5}\,dx}}\\ & = \left. {\left( {\frac{1}{2}{x^6} - {x^3} - \frac{3}{2}{x^2} + 3x} \right)} \right|_{ - 1}^1 + \left. {\left( {12x + 24{x^2} - {x^3} - \frac{1}{2}{x^6}} \right)} \right|_{ - 2}^2\\ & = \hspace{1.15in}4\hspace{1.4in} + \hspace{1.15in}32\\ & = \require{bbox} \bbox[2pt,border:1px solid black]{{36}}\end{align*}\]

Don’t always expect every integral over a region to be done with a single integral. On occasion you will need to split the integral up and do the actual integration over separate sub regions. In this case that was obvious but sometimes it might not be so clear until you get into the problem and realize it would be easier to do over sub regions.